-
北京西郊林场总面积为60.59 km2,包含多个风景名胜点和森林公园,周边环绕着多处党政军民单位,特殊的地理位置决定了护林防火是林场森林资源管护工作的重中之重。每年防火期林场需要投入大量人财物力,布设巡航路线、火源检查卡点、火灾监测点以预防和监控林火发生。过去管理者主要通过经验安排防火监测点,大部分监测是由人工巡视、瞭望完成,监测效率低。随着经济发展和智慧林业的兴起,林场拟建立全天候的林火监测体系,以提高林区监测的效率。基于瞭望塔的林火监测网是智慧林业“地网”感知层的重要基础设施[1],对生态保护、防火救灾、森林公园的区域安全都具有重要意义[2-5]。建立林区瞭望塔监测体系已成为当前研究热点[6-7]。受地理空间环境与投资总成本约束,林区瞭望塔应合理布局、统一规划,从而实现监测覆盖面积最大化。为满足林区瞭望塔建设空间可视性的最大化[8],需借助地理信息系统(GIS)构建选址模型,设计相应的算法,优化瞭望塔的布局。受林区瞭望塔建设耗时且具有明显阶段性和动态性的制约,应进一步研究近似最优布局中各瞭望塔建设时序,以优化监测网的阶段效益。除监测(可视)面积外,交通、坡度、已有建筑等也影响瞭望塔建设时序的合理性。即时序优化问题是典型的多属性排序问题,常用层次分析法、模糊综合评判法求解[9-13]。本研究收集林场的1∶10 000等高线地形图、防火公路图、含风景点的建筑设施图等数据,以顶点法提取瞭望塔备选点集,根据备选点集建立最大监测面积模型(maximum monitoring area model,Max-MAM),利用启发算法中复杂度较低且便于与GIS结合的Greedy算法,快速求解近似最优布局方案,以优化监测网整体监测效率;利用熵权法建立动态多属性建设时序模型(dynamic multi-attribute construction time sequencing model,DMACTSM),对瞭望塔建设时序进行动态优化布局,以提高瞭望塔建设效益。
-
最大监测面积模型(Max-MAM)可表述为从备选点集P中选择n个点,使得n个点构成监测网络的监测(可视)面积最大。设选择n个点建成的监测网为
$ {\overline{P}}_{n} $ ={p1,p2,$\cdots $ ,pn}。其中,pn为第n个备选点选建的瞭望塔;Sn(p1,p2,$\cdots $ ,pn)为$ {\overline{P}}_{n} $ 的监测面积。则最大监测面积模型为:$$ S_{n}^*(p_1^*,p_2^*, \cdots ,p_{n}^*) = {\rm{Max}}\;{S_{\!\!n}}({p_1},{p_2}, \cdots ,{p_n})\text{。} $$ (1) 由式(1)可得
$ {\overline{{P}}}_{{n}}^* $ ={$ {p}_{1}^{*} $ ,$ {p}_{2}^{*} $ ,$\cdots $ ,$ {p}_{n}^{*} $ }即为最优布局,此时n值为根据约束(如投资)或监测网预设目标确定的待建瞭望塔数;$ {p}_{i}^{*} $ 为第i次选建时的最优瞭望塔;$ {{S}}_{{n}}^* $ 为$ {\overline{P}}_{n} $ 的最大监测面积。Greedy算法结合GIS视域分析工具可实现Max-MAM的快速近似求解。记Pi={
$ {p}^{i}_{j} $ ,j=1,$\cdots $ ,i,$ {p}^{i}_{j} $ ∈P}为第i次迭代时备选点集;$ {\overline{P}}_{i} $ ={$ {{p}^{i}_{j}}^{*} $ ,j=1,…,N−i,$ {{p}^{i}_{j}}^{*} $ ∈P}为第i次迭代时已选瞭望塔集,满足式(2)关系,其中0≤i≤n≤N。$$ {P_0} = P,\;{\overline P _0} = {\text{Ø}} ,\;{P_i} \cup {\overline P _i}{\rm{ = }}P{\text{且}}{P_i} \cap {\overline P _i} = {\text{Ø}} \text{。} $$ (2) 根据上述定义,Greedy算法第i次迭代选择的最优备选点
$ {{p}_{i}^{i}}^{*} $ 可表示为:$$ S_i^*({{p_1^i}^*},{{p_2^i}^*}, \cdots ,{{p_{i - 1}^i}^*},{{p_i^i}^*}) = {\rm{Max}}\;{S_i}({{p_1^{i - 1}}^*},{{p_2^{i - 1}}^*}, \cdots ,{{p_{i - 1}^{i - 1}}^*},p_i^i),\;{\text{其中}}p_i^i \in {\rm{P}}\text{。} $$ (3) 式(3)中,
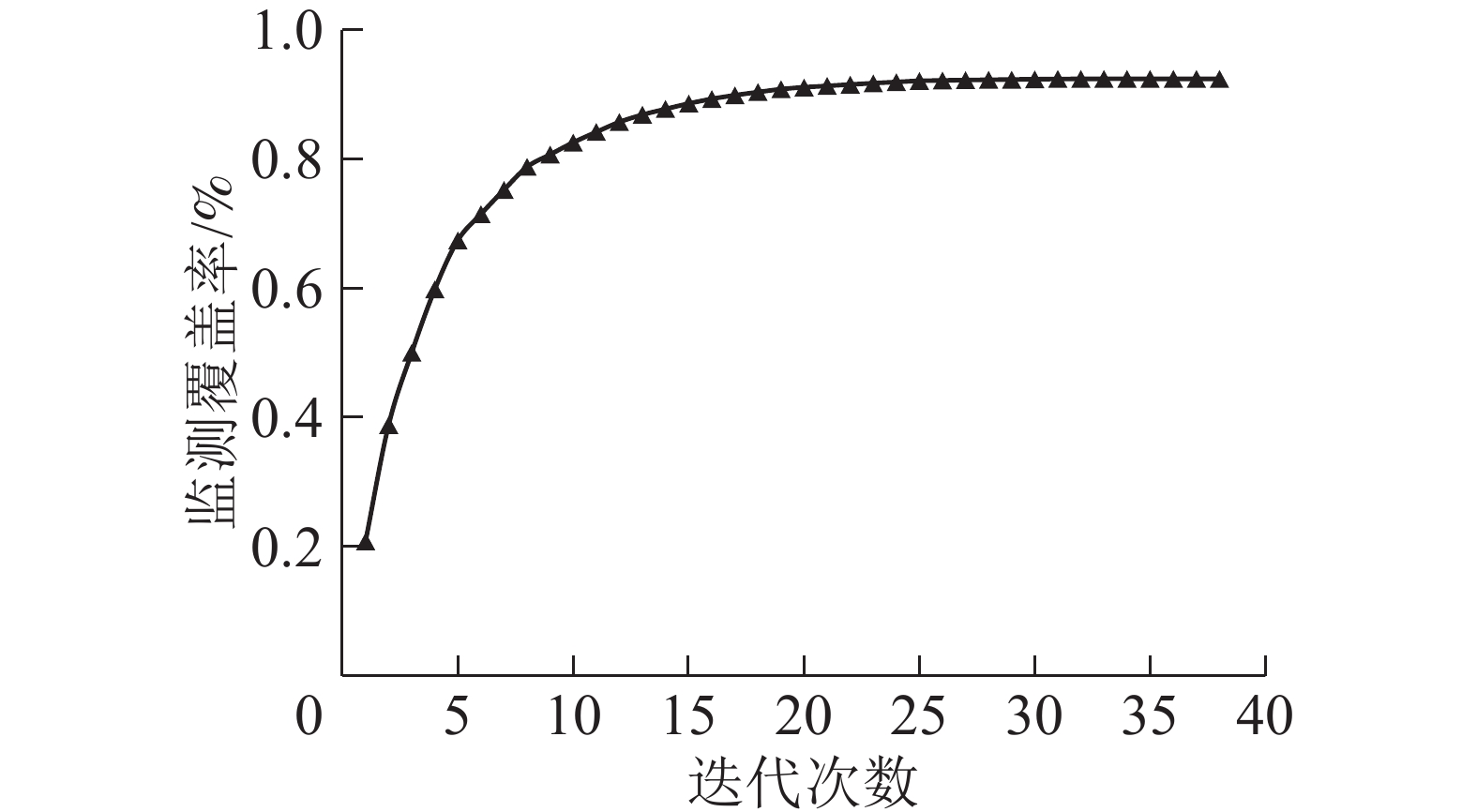
$ {S}_{i} $ (p1,p2,…,pi)是监测网$ {\overline{P}}_{i} $ 的监测面积(可视域面积),由GIS视域分析工具统计$ {\overline{P}}_{i} $ 可视域栅格数$ \left|{V}_{\rm{i}}\right| $ 得到。结合ArcPy中的visibility_3d函数运行上述Greedy算法迭代计算可得最大监测面积$ {S}_{i}^{*} $ ($ {{p}_{1}^{i}}^{*} $ ,$ {{p}_{2}^{i}}^{*} $ ,…,$ {{p}_{i}^{i}}^{*} $ )。Greedy算法应用于W林场38个瞭望塔备选点,监测覆盖率随迭代次数变化如图2所示。表1列出了Greedy算法前6次迭代得到的瞭望塔优化布局方案,当i=5时,优化布局方案
$ {\overline{{P}}}_{{6}} $ ={$ {{p}}_1^* $ ,…,$ {{p}}_{{6}}^* $ } ={a6,a29,a36,a12,a26,a18},结合图1可知,$ {\overline{{P}}}_{{6}} $ 的监测覆盖率达71.47%,近似实现了设计最少的瞭望塔数目及空间布局、以获得最大监测覆盖面积的整体优化目标。表 1 布局方案与可视栅格总数变化
Table 1. Layout scheme and the change of the total number of visible grids
i $ {\overline{{P}}}_{{i}} $ ${\left|{{V}}_{{i}}\right| }$ 0 6 1 972 1 6,29 3 677 2 6,29,36 4 737 3 6,29,36,12 5 664 4 6,29,36,12,26 6 384 5 6,29,36,12,26,18 6 767 -
为进一步提升瞭望塔网络建设过程的阶段性效益,建立DMACTSM模型,通过动态计算布局方案中各瞭望塔的综合属性值,对
$ {\overline{P}}_{n} $ 中各瞭望塔的建设时序进行优化分析。设$ {u}_{i}^{k} $ 为第k次迭代计算瞭望塔$ {p}_{i}^{k} $ 的综合属性值,则第k次迭代得到优先建设的瞭望塔$ {{p}_{i}^{k}}^{*} $ 满足如下最大化问题:$$ {{u}_{i}^{k}}^* = \max \{{u}_{i}^{k}\}\text{。} $$ (4) 式(4)中:
$ {{p}_{i}^{k}}^* $ ,$ {p}_{i}^{k} $ ∈$ {\overline{P}}_{n} $ (k=0,1,$\cdots $ ,n−2),则监测网$ {\overline{P}}_{n} $ 的最优建设时序为$ {{p}_{i}^{0}}^{*} $ 、$ {{p}_{i}^{1}}^{*} $ 、$\cdots $ 、$ {{p}_{i}^{n-1}}^{*} $ 。MACTSM对建设时序的优化包括3步。 -
新建塔要求交通便利,地势平坦,尽量依附已有建筑建设[7]。表2给出了MACTSM的4个属性,增长监测面积、交通情况、坡度与依附建筑情况。记pi的增长监测面积为yi1,指加入
$ {p}_{i} $ 后$ {\overline{P}}_{i-1} $ 增加的监测面积,即pi对监测网$ {\overline{P}}_{i} $ 的单独监测面积[8]。 yi1是正向属性,反映新增瞭望塔对监测网总监测面积的贡献,随监测网变化而变化,满足$ {y}_{i1}{=}\left(\left|{{\rm{V}}}_{{i}}\right|{=}\left|{{\rm{V}}}_{{i}{-}{1}}\right|\right){×}{s} $ ,其中,$ \left|{V}_{i}\right| $ 是监测网$ {\overline{P}}_{i} $ 可视域栅格数,s是单位栅格面积。设交通情况指瞭望塔所处位置距离道路的远近,即瞭望塔到最近道路的垂线长度(m),对林区防火公路图进行近邻分析求得。坡度指林区地面的陡峭程度(°),对DEM进行坡度分析求得。依附建筑情况指瞭望塔与已有建筑的贴近程度,即瞭望塔距最近已有建筑的直线距离(m),对林区含风景点的建筑设施图进行近邻分析求得。该决策矩阵可记为Y=(yij)n×4,其中,j为属性编号,yi=(yi1,$\cdots $ ,yi4)为瞭望塔$ {p}_{i}^{*} $ 的属性向量,i=1,2,$\cdots $ ,n。表 2 属性集
Table 2. Attribute set
属性 属性名称 属性类型 yi1 增长监测面积 正向、收益、动态 yi2 交通情况 反向、成本、静态 yi3 坡度 反向、成本、静态 yi4 依附建筑情况 反向、成本、静态 根据式(5)对正向属性yi1消除量纲,对反向属性yi2、yi3、yi4进行极差标准化,再进行归一化,得到决策矩阵Z=(Zij)n×4。
$$ \left\{ {\begin{aligned} & {y_j^* = {{\max }_{1 \leqslant i \leqslant n}}{y^{}_{ij}}},\\ & {y_j^0 = {{\min }_{1 \leqslant i \leqslant n}}{y^{}_{ij}}},\\ & {{x^{}_{ij}} = \frac{{{y^{}_{ij}} - y_j^0}}{{y_j^* - y_j^0}}}; \end{aligned}} \right.\;\;\left\{ {\begin{aligned} & {y_j^0 = {{\max }_{1 \leqslant i \leqslant n}}{y^{}_{ij}}},\\& {y_j^* = {{\min }_{1 \leqslant i \leqslant n}}{y^{}_{ij}}},\\& {{x^{}_{ij}} = \frac{{y_j^0 - {y^{}_{ij}}}}{{y_j^0 - y_j^*}}}; \end{aligned}} \right. \;\; {{Z}}^{}_{{ij}}{=}\frac{{{x}}^{}_{{ij}}}{\sum\limits _{{i}{=1}}^{n}{{x}}_{{ij}}}\text{。}$$ (5) -
对归一化矩阵Z采用熵权法确定权重。熵权法不依赖于专家经验,可通过数据间差异客观确定属性权重[16]。根据式(6)分3步进行确权:先计算各属性熵值ej;然后计算各属性差异系数gj;最后计算各属性权重ωj。
$$ {{e}}^{}_{{j}}{=}{-}{k}\sum _{{i}{=}{1}}^{{n}}{{Z}}_{{ij}}{\ln}{{Z}}_{{ij}}{,}\;{\text{其中}},\;k=\frac{1}{{\ln}\;n}{\text{。}}{{g}}^{}_{{j}}{=}{1}{-}{{e}}^{}_{{j}} {\text{。}}\omega_{{j}}{=}\frac{{{g}}^{}_{{j}}}{\displaystyle\sum\limits _{{j}{=}{1}}^{{4}}{{g}}^{}_{{j}}}\text{。} $$ (6) 若各属性已有主观权重时,则可采用式(7)修正。建设前期注重增长监测面积可以将yi1权值提高,当yi1权值为1,表示不关心其他属性,排序结果与Greedy算法一致。
$$ \omega_{{j}}^{{0}}{=}\frac{\lambda_{{j}}\omega_{{j}}}{\displaystyle\sum\limits _{{j}{=}{1}}^{{4}}\lambda_{{j}}\omega_{{j}}}\text{。} $$ (7) -
根据式(8)对各属性线性加权,得瞭望塔pi综合属性值。
$$ {{u}}_{{i}}{=}\sum _{{j}{=}{1}}^{{4}}\omega_{{j}}{{x}}_{{ij}}\text{。} $$ (8) 以林场优化布局方案
$ {\overline{P}}_{6} $ =$ \{{p}_{1}^*,\dots ,{p}_{6}^*\} $ ={a6,a29,a36,a12,a26,a18}为例。当k=0时,对林区DEM数据、防火公路图、建筑设施(含风景点)图等数据进行领域分析计算,6个瞭望塔4个属性的初始决策矩阵Z0。$$ {{{Z}}^0} = \left[\!\!\! {\begin{array}{*{20}{l}} {0.43}&{0.28}&{0}&{0}\\ {0.27}&{0.22}&{0.32}&{0.22}\\ {0.05}&{0.28}&{0.02}&{0.22}\\ {0.22}&{0.19}&{0.11}&{0.10}\\ {0.03}&{0}&{0.25}&{0.25}\\ {0}&{0.03}&{0.29}&{0.20} \end{array}} \!\!\!\right]\text{。} $$ 计算可知:6个瞭望塔的综合属性值向量u0=(0.67,0.76,0.35,0.50,0.30,0.29)。
$ {{u}}_2^{{0}} $ =max{$ {{u}}_{{i}}^{{0}} $ }=0.76,$ {{p}}_2^* $ (即$ {{a}}_{{29}} $ 瞭望塔)率先被加入建设序列。同理,当k=1时,对余下5个瞭望塔重新计算增长监测面积属性值,得到归一化决策矩阵Z1,计算可知:第2个被加入建设序列的瞭望塔为
$ {{a}}_{{26}} $ ;依此类推,DMACTSM迭代5次,得到各阶段被优先建立的瞭望塔及最终建设时序优化结果,如表3所示。表 3 基于DMACTSM迭代计算的综合属性值、选优和最终排序
Table 3. DMACTSM iterative computation
迭代次数 综合属性值 max(ui) 优建瞭望塔 a29 a26 a12 a6 a36 a18 0 0.76 0.30 0.50 0.68 0.35 0.29 0.76 $ {{a}}_{{29}} $ 1 0.56 0.45 0.55 0.47 0.44 0.56 $ {{a}}_{{26}} $ 2 0.54 0.51 0.36 0.50 0.54 $ {{a}}_{{12}} $ 3 0.54 0.33 0.48 0.54 $ {{a}}_{{6}} $ 4 0.53 0.47 0.53 $ {{a}}_{{36}} $ 林场瞭望塔网络优化布局及建设时序效果,如图3所示。对比图4、图5可得:监测覆盖面积增长最快的建设时序为a6、a29、a36、a12、a26、a18,DMACTSM调整后建设时序为a29、a26、a12、a6、a36、a18,不仅保证瞭望塔网络总体效益最优,也实现了各阶段建设效益的优化,说明DMACTSM对瞭望塔建设过程优化有实际意义。

图 3 W林场瞭望塔网络优化设计(最优布局及建设时序)
Figure 3. Optimal design of watchtower network in W (optimal layout and construction sequence)

图 4 优化前后标准化增长监测面积对比
Figure 4. Comparison of standardized growth monitoring area before and after optimization

图 5 优化前后综合属性值对比
Figure 5. Comparison of comprehensive attribute values before and after optimization
雷达图可对比优化前后首建瞭望塔的效益(图6)。首建
$ {{a}}_{{6}} $ 增长监测面积最大,在其他成本属性和综合值上均不如率先建立$ {{a}}_{{29}} $ 。 -
林区瞭望塔网络建设具有明显的空间性、阶段性和动态性。本研究结合林场实际,将林区瞭望塔网络建设规划问题转换为瞭望塔布局与时序优化问题,建立了最大监测面积模型(Max-MAM)和动态多属性建设时序模型(DMACTSM),并利用GIS工具设计实现了求解模型的方法。计算和实际验证表明:Max-MAM及Greedy算法可得到满意的优化布局方案;DMACTSM兼顾收益与多个成本属性,在瞭望塔建设时序的综合优化中兼顾了瞭望塔建设的整体与阶段效益的优化,且计算方法实用可行,为林区瞭望塔网络建设规划提供科学依据。
研究发现:顶点法提取备选点集是优化的基础,其关键是确定提取窗口的尺寸[15]。本研究采用11×11栅格大小的窗口提取备选点集,窗口尺寸可通过试验计算进一步优化提高顶点提取的效度。监测覆盖率最大与瞭望塔数最小问题互为对偶,当预设约束不是瞭望塔数n,而是监测覆盖率时,求解Max-MAM同样可得近似最优方案。本研究选取了面积较小的林场作为方法研究实验区,所提出的时空优化设计方法与研究对象的面积大小无关。在实践中,对于面积大的林区,甚至是1个县、1个州市的林区,更能体现时空优化设计带来的经济效益。
Layout and optimal design of watchtowers in forest regions
-
摘要:
目的 为优化林区瞭望塔布局与建设时序,提高瞭望塔网络建设效率。 方法 以北京郊区某林场为实验点,针对瞭望塔网络建设的空间性、阶段性和动态性等特点,设计基于顶点法的最大监测面积模型(Max-MAM),利用Greedy算法求解近似最优布局方案;设计基于熵权法的动态多属性建设时序模型(DMACTSM),求解近似最优布局方案下的综合最优建设时序。 结果 顶点法共提取到38个备选点;用Max-MAM优选出最优布局方案 $ {\overline P_6} = \left\{ {{a_6},{a_{29}},{a_{36}},{a_{12}},{a_{26}},{a_{18}}} \right\}$ ,可使瞭望塔网络的监测覆盖面积最大,约71.47%;用DMACTSM模型获得最优建设时序为a29、a26、a12、a6、a36和a18,可使瞭望塔网络建设过程中综合效益最优。结论 Max-MAM和DMACTSM能够调整建设时序,解决建设时序最优问题,结合地理信息系统(GIS)实现瞭望塔网络布局优化;调整后的建设时序在增长监测面积、交通、坡度、已有建筑情况等方面综合最优。图6表3参16 Abstract:Objective With the optimization of tower layout and time sequence in the process of construction, this paper is aimed at the promotion of the construction efficiency of tower networks in forest regions. Method With one forest farm in the suburbs of Beijing chosen as the testing spot and features of watchtower network construction such as spatiality, periodicity and dynamicity taken into consideration, efforts were made to figure out a near-optimal layout design built employing Greedy algorithm on the basis of Maximum monitoring area model(Max-MAM) established with the vertex method. Meanwhile, with such a design, on the basis of DAMACSTM which was built employing the dynamic multi-attribute entropy weight method, the optimal time sequence of construction was figured out. Result 38 optional points extracted adopting the vertex method, with the optimal layout achieved by Max-MAM, $ {\overline {P}_6} = \left\{ {{a_6},{a_{29}},{a_{36}},{a_{12}},{a_{26}},{a_{18}}} \right\}$ , the monitoring coverage of the watchtower network was maximized (about 71.47%) while with the optimal time sequence of construction achieved with DAMACSTM, a29>a26>a12>a6>a36>a18, the comprehensive benefits are maximized.Conclusion Two models of Max-MAM and DMACTSM proposed in this paper, can adjust and figure out the optimal time sequence of construction and when combined with GIS, are conducive to the achievement of the optimal layout of watchtower networks. And the optimal time sequence of construction helps maximize the comprehensive benefits in aspects like the increase in the monitoring area, the traffic, the slope, and existing structures. [Ch, 6 fig. 3 tab. 16 ref.] -
Key words:
- forest engineering /
- forest watchtower layout /
- Max-MAM /
- DMACTSM /
- entropy weight method /
- Greedy algorithm
-
香榧Torreya grandis ‘Merrillii’是红豆杉科Taxaceae榧树属Torreya的优质品种,其种子可食用,是著名的特色干果[1];种仁含油率较高[2],具有很高的经济价值[3]。随着各类栽培技术的开发,中国的香榧种植产业规模也在不断扩大,对于香榧植株及干果[4]的有关研究已经比较成熟,但对于香榧愈伤组织培养的研究还处在相对落后的阶段。
植物愈伤细胞的悬浮培养是一种通过调控细胞生长,快速获得大量细胞以及目的产物的技术,不受各类局限进行周年生产。建立好的培养体系以及优化培养条件,可以超过原有的生产方式[5]。王沐兰等[6]通过建立红豆杉Taxus chinensis高产悬浮细胞系,提高了紫杉醇的产量;赵文佳[7]诱导青钱柳Cyclocarya paliurus红色愈伤系,并利用细胞悬浮培养技术建立了花青素的生产体系。此外,国槐Sophora japonica[8]、南方红豆杉Taxus wallichiana[9]和落叶松Larix gmelinii[10]等植物的悬浮培养体系也已经建立。然而在香榧悬浮培养体系中,细胞生长动力学规律及最佳培养条件尚不清晰。
悬浮细胞的培养过程中需添加各类激素以促生长。赤霉素(GAs)是植物体内的一种重要激素,已被发现较多的种类,并按顺序命名为赤霉素A1 (GA1)、赤霉素A3 (GA3)等,在植物生长的各个环节都不可缺少[11−12]。在非生物胁迫或生物刺激下,外施赤霉素可以增加防御酶的数量或活性,进而影响植物抗性[13]。赤霉素还可对植物愈伤组织增殖过程中的褐化情况进行抑制,促进愈伤组织增殖[14]。
鉴于此,本研究通过优化香榧胚性愈伤组织悬浮培养基,探究其动力学特性,筛选最适合的培养条件,同时探究GA3处理下香榧胚性愈伤组织生长及差异基因表达的规律,为香榧愈伤组织的生长和遗传转化体系的构建提供科学依据。
1. 材料与方法
1.1 材料及培养条件
以浙江农林大学省部共建亚热带森林培育国家重点实验室培育的香榧胚性愈伤组织为试验材料。使用SH液体培养基,其中添加脱落酸(ABA) 1.0 mg·L−1、水解酪蛋白(CH) 500.0 mg·L−1、蔗糖30.0 g·L−1、活性炭2.0 g·L−1和GA3 0.5 mg·L−1,设置pH 5.7,摇床转速为110 r·min−1,25 ℃暗培养。
1.2 悬浮培养细胞生长曲线及活力测定
在100 mL的锥形瓶中添置30 mL的SH液体培养基(配方同1.1),香榧胚性愈伤组织接种量为30 g·L−1,设置pH 5.7,转速为110 r·min−1,每3 d取样测定细胞鲜质量及细胞活力(氯化三苯四氮还原法,TTC),即波长为485 nm处的吸光度。
1.3 悬浮培养条件优化
1.3.1 培养条件筛选
在100 mL锥形瓶中添置30 mL的SH液体培养基(配方及培养条件同1.1),设置转接愈伤组织接种量为10、20、30、40、50 g·L−1;pH为5.5、5.6、5.7、5.8、5.9;摇床转速为70、90、110、130、150 r·min−1。25 ℃摇床中振荡暗培养。每个处理设置3个重复,21 d时统计鲜质量,计算细胞增长率,细胞增长率=(收获量−接种量)/接种量×100%。
1.3.2 抗氧化酶活性测定
超氧化物歧化酶(SOD)活性采用氮蓝四唑光化还原法测定;过氧化物酶(POD)活性采用愈创木酚法测定;过氧化氢酶(CAT)活性采用紫外吸收法测定[15]。
1.4 测序RNA提取
将香榧胚性愈伤组织接种于SH液体培养基中(配方及培养条件同1.1),设置对照组(ck)和实验组(GA3+),对照组培养基中不添加GA3,实验组培养基中施加GA3。18 d后收集材料,使用多糖多酚植物总RNA提取试剂盒(北京天根生化科技有限公司),按说明书提取RNA,分别设置3个生物学重复,使用分光光度计检测浓度与纯度。
1.5 转录组测序
测序工作由北京诺禾致源科技有限公司完成,采用Illumina HiSeqTM 2000测序平台进行测序。由于香榧没有参考基因组,将聚类分析结果中最长的转录本作为参考序列单基因簇进行后续分析。
1.6 差异表达基因的筛选及功能注释
采用RSEM软件将每个样品过滤后的数据(clean reads)在参考序列上做比对。采用DESeq分析差异表达基因(DEG),以|log2Fc|>1 (Fc为表达量的差异倍数)且P<0.005为筛选条件[16]。将差异表达基因注释到基因本体论数据库(GO)和京都基因与基因组百科全书(KEGG),并进行GO和KEGG富集分析。
1.7 实时荧光定量PCR (RT-qPCR)验证
使用Primer 3.0 input在线软件设计引物(表1),以TgActin为内参基因[16]。用Takara生物科技有限公司的反转录试剂将提取的RNA反转录成cDNA进行RT-qPCR验证,每个样品3个生物学重复。反应体系为10.0 μL,包括5.0 μL的TB GREEN,0.2 μL的上下游引物,0.4 μL的模板cDNA,4.2 μL的ddH2O。PCR反应程序为:95 ℃ 10 min,95 ℃ 10 s,60 ℃ 1 min,95 ℃ 30 s,60 ℃ 15 s共39个循环。
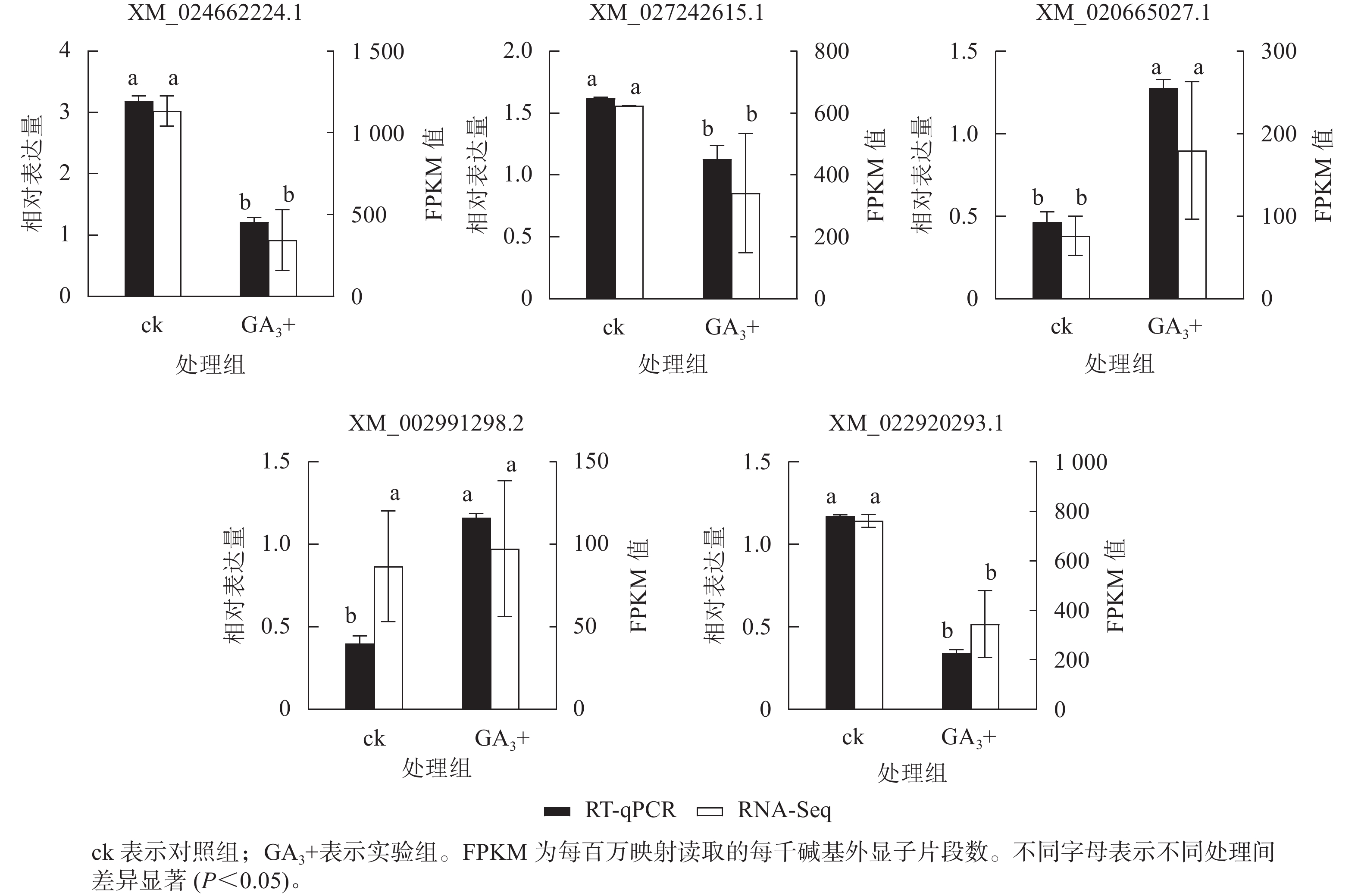
表 1 差异表达基因RT-qPCR的特异性引物信息Table 1 Gene-specific primer information for RT-qPCR引物名称 正向引物(5′→3′) 反向引物(5′→3′) XM_024662224.1 TCCAAGGGAAGGGGAACATC TCCCCGGATTGCAGAAGATT XM_027242615.1 TGTACCCTCCACCCTTTTCC TCTATCAGGGAGGGAGCAGA XM_020665027.1 TACGACCAGTCAGAGGCTTG AAACACCCACCGTCTTTGAC XM_002991298.2 CACGCCCAATTTTCACGAGA CGAAAACTAGGCAGGGCATC XM_022920293.1 TCGACCTTGAGACCTGGAAC TGATGGTGCAGCAAATCAGG TgActin TGGCATCTCTCAGCACATTCCA TGCCAACATCTCAAGCAAGCAC 1.8 数据分析
使用Excel处理数据,采用SPSS 26进行单因素方差分析(one-way ANOVA)和多重比较(邓肯法),显著性水平为0.05,使用GraphPad Prism 9软件作图。
2. 结果与分析
2.1 香榧胚性愈伤组织悬浮培养的动力学
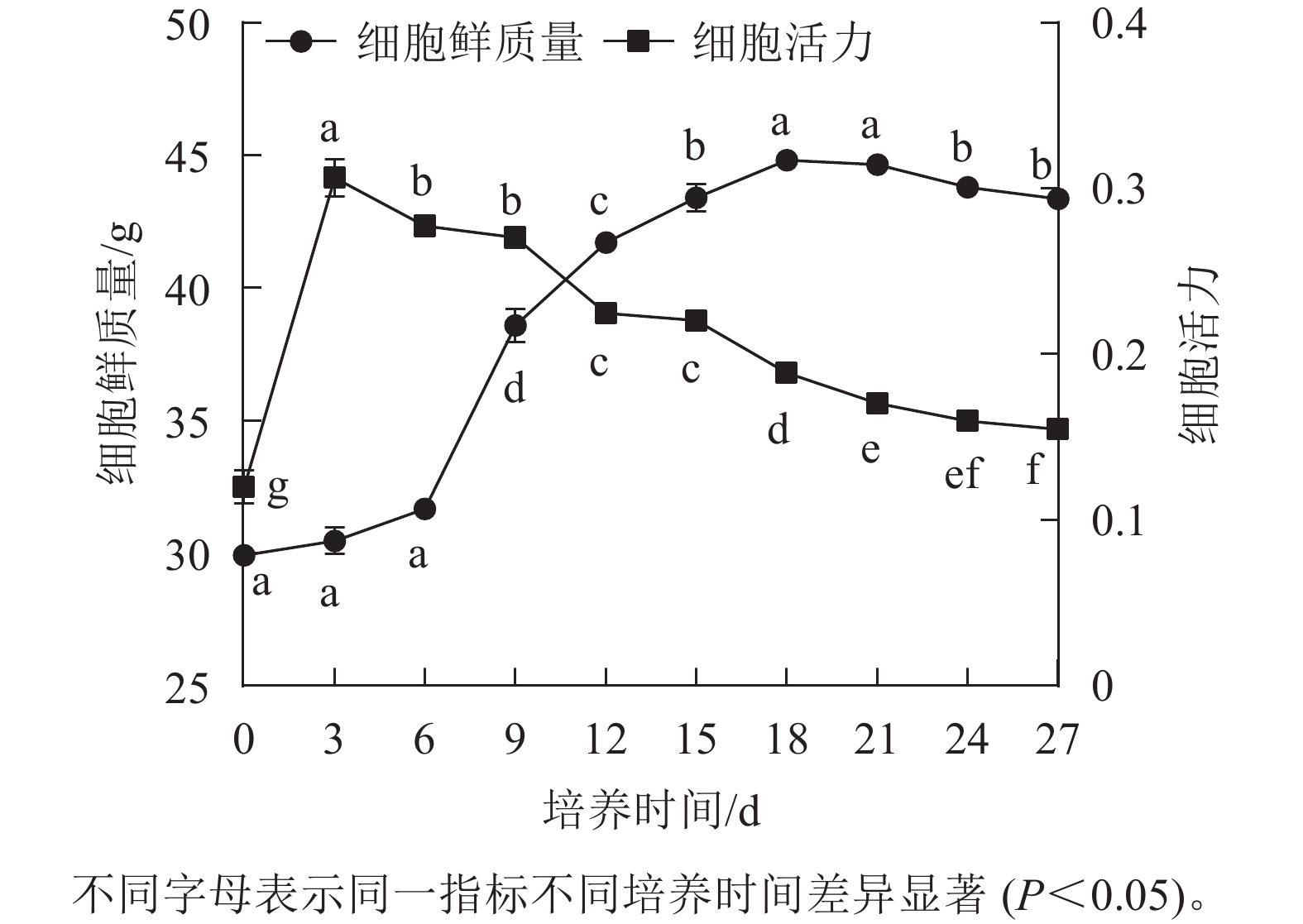
香榧胚性愈伤组织悬浮培养时,细胞鲜质量变化呈“S”型曲线。在0~6 d鲜质量增加缓慢;7~18 d鲜质量快速增加;18 d时达到最高值,为44.81 g;18~21 d进入稳定期,鲜质量基本不再增加;21 d后细胞进入衰退期,鲜质量下降。同时,在培养周期内香榧胚性细胞活力在0~3 d时快速增长,并在3 d时达到最高值(0.32),之后持续下降,并且随着培养时间的延长,变化趋势趋向平缓(图1),表明9~12 d为香榧胚性愈伤组织悬浮培养的最佳继代时期。
2.2 香榧胚性愈伤组织悬浮培养条件优化
2.2.1 接种量对香榧悬浮细胞的影响
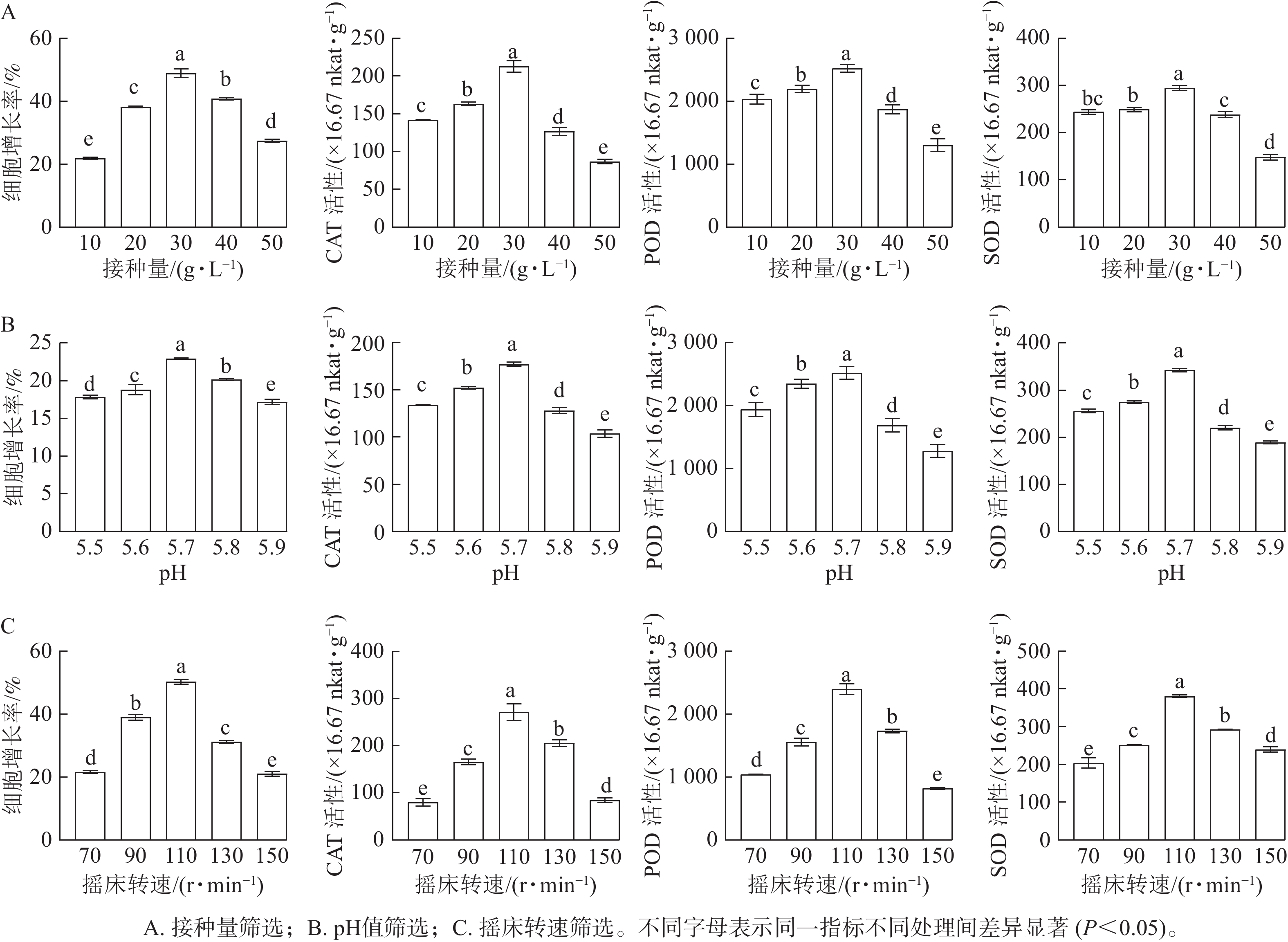
随着接种量的增加,胚性愈伤组织增长率呈先上升后下降的趋势。当愈伤组织接种量为10 g·L−1时,细胞增长率最低(21.78%);当接种量为30 g·L−1时,细胞增长率显著升高(P<0.05),达到最高值,为49.94%。SOD、POD和CAT活性均随接种量的增加而呈先升高后降低的趋势。当接种量为30 g·L−1时,SOD、POD和CAT活性均最高,显著高于其他接种量时的酶活性(P<0.05)(图2A)。
2.2.2 pH对香榧悬浮细胞的影响
随着pH的增加,香榧悬浮细胞增长率呈先上升后下降的趋势。当培养基pH为5.7时,细胞鲜质量增殖效果最佳,细胞增长率最大,为22.95%,显著高于其他处理(P<0.05)。SOD、POD和CAT活性均随接种量的增加而呈先升高后降低的趋势。在pH为5.7时,悬浮细胞SOD、POD和CAT活性均最大,显著高于其他处理(P<0.05)(图2B)。
2.2.3 转速对香榧悬浮细胞的影响
随着转速的增加,香榧悬浮细胞增长率呈逐渐上升的趋势,当转速为70 r·min−1时,细胞增长率较低,为20.33%;当转速达110 r·min−1时,细胞增长率最大,为50.84%,显著高于其他处理(P<0.05)。SOD、POD和CAT活性均随摇床转速的增加而呈先升高后降低的趋势。当转速为110 r·min−1时,SOD、POD和CAT活性最大,显著高于其他处理(P<0.05)(图2C)。
2.3 外源GA3对香榧胚性愈伤组织的影响
2.3.1 外源GA3处理下香榧胚性愈伤组织的转录组测序分析
所有检测样品纯净碱基数均达6.66 Gb以上,质量为Q20 (每项碱基质量大于20的碱基数占总碱基数的比例)的碱基比例均大于97.47%,达Q30 (每项碱基质量大于30的碱基数占总碱基数的比例)以上的碱基比例大于92.70%,每个样本碱基GC含量为43.53%~44.45%,较为一致,且测序碱基平均错误率均在0.1%以下,表明转录组数据较为准确。
2.3.2 差异表达基因分析
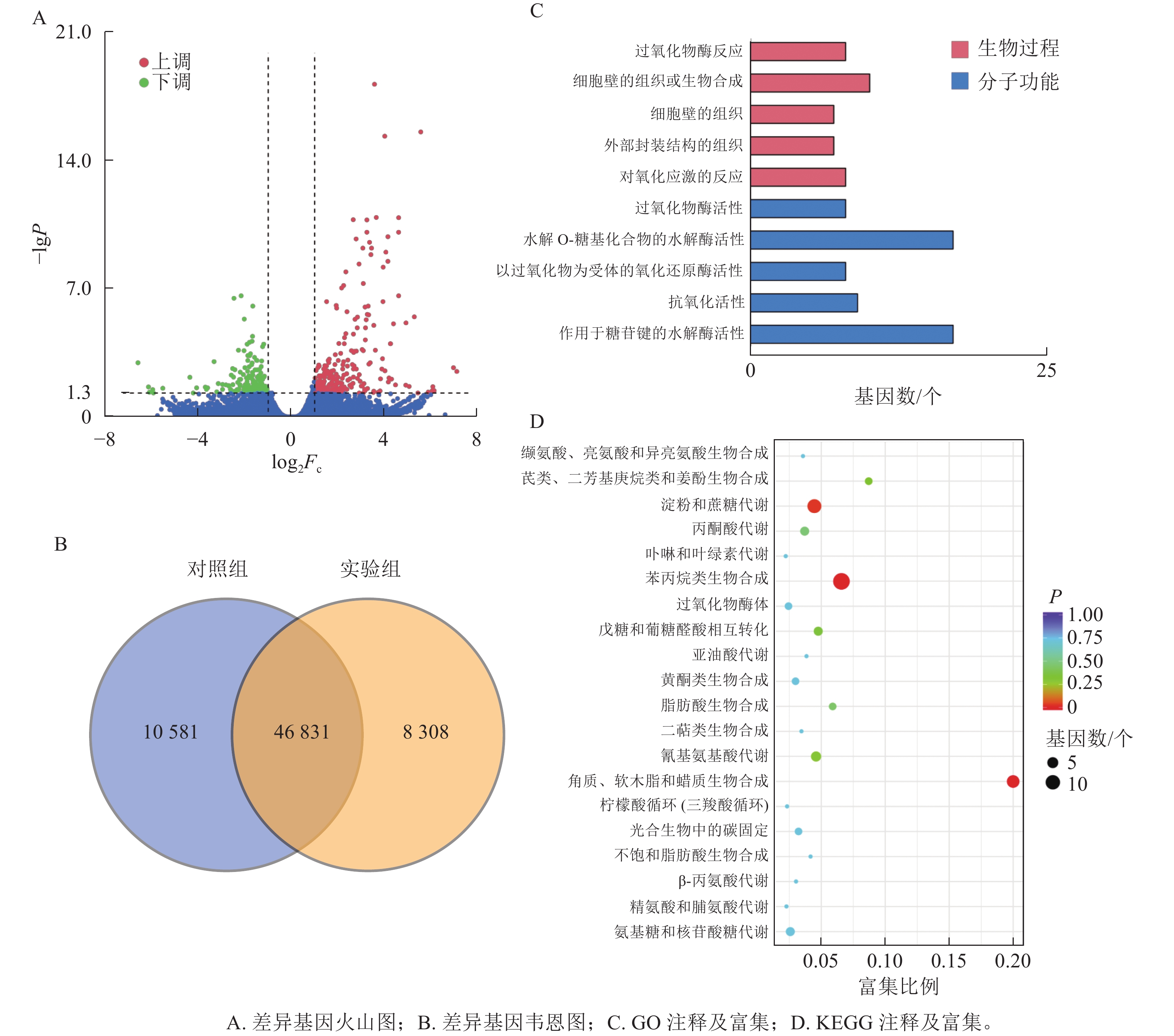
本研究共筛选到428个差异表达基因,其中236个基因上调表达,192个基因下调表达(图3A)。对照组与实验组共有表达的基因数为46 831个,对照组特有表达的基因数为10 580个,实验组特有表达的基因数为8 308个(图3B)。
2.3.3 差异基因GO注释及富集分析
GO功能注释与分类统计发现:差异表达基因被归类到2个功能分类组中,其中:分子功能中有17个基因涉及水解邻糖基化合物的水解酶活性;生物过程中细胞壁组织或生物合成过程注释到10个差异基因(图3C)。
2.3.4 差异基因KEGG注释及富集分析
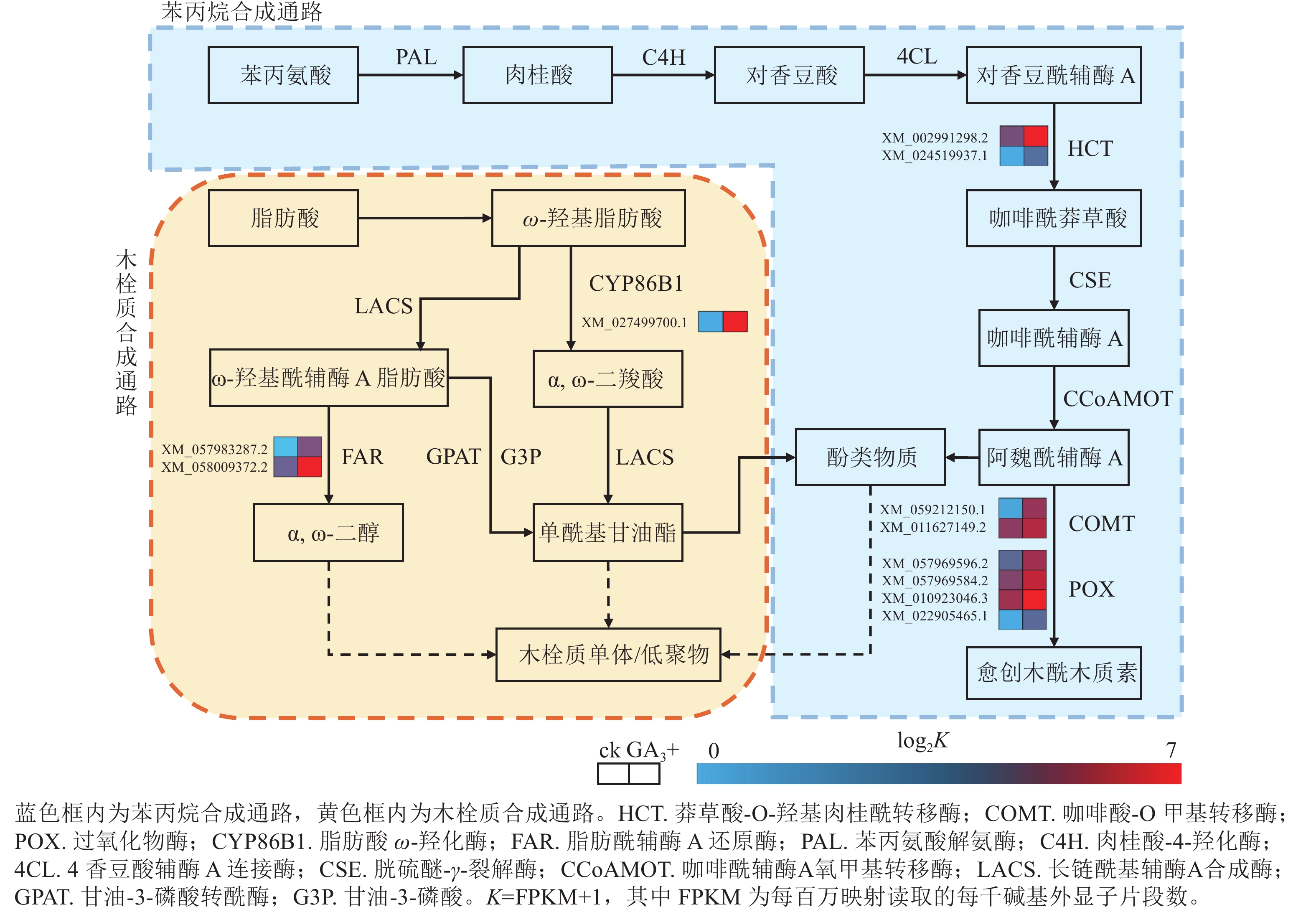
将差异表达基因进行KEGG通路富集分析,对富集较多的前20个通路进行散点图绘制。差异基因富集最多的通路为苯丙烷生物合成;其次为淀粉和蔗糖代谢;再次为角质、木栓质和蜡的生物合成。而氰基氨基酸代谢、戊糖和葡萄糖醛酸相互转化、丙酮酸代谢、氨基糖和核苷酸糖代谢等通路富集较少(图3D)。
本研究有8个与苯丙烷生物合成相关的差异基因被富集到且呈上调表达,4个与过氧化物酶(POX)相关;2个与莽草酸-O-羟基肉桂酰转移酶(HCT)相关;2个与咖啡酸-O甲基转移酶(COMT)相关(图4)。本研究表明:GA3处理会使得香榧胚性愈伤组织细胞增加对苯丙氨酸的合成与利用,调控HCT、COMT、POX活性的基因上调表达,促进了对香豆酰辅酶A参与合成咖啡酰莽草酸、愈创木酰木质素等产物的过程,同时生成酚类物质参与其他途径物质合成。该过程产生的酚类物质还可能参与清除部分愈伤组织和培养基产生的有害活性氧,提高香榧胚性愈伤组织的抗性[17−19]。
本研究有3个与木栓质生物合成相关的差异基因被富集到且呈上调表达,其中1个与脂肪酸ω-羟化酶(CYPB6B1)相关,2个与脂肪酰辅酶A还原酶(FAR)相关(图4)。与对照组相比,GA3处理会使FAR与CYPB6B1上调表达,参与ω-羟基脂肪酸下游α,ω-二羧酸和α,ω-二醇合成途径,促进下游单酰基甘油酯的合成,共同参与形成木栓质单体或低聚物,同时产生酚类物质[20]。该过程可结合苯丙烷合成途径产生的酚类物质,促进木栓质单体或低聚物的合成,应对环境变化并进行调整[21−22]。
2.3.5 GA3处理下香榧胚性愈伤组织差异表达基因RT-qPCR验证
RT-qPCR验证结果(图5)表明:5个基因与转录组测序结果呈现相同的表达趋势,2个基因上调表达,3个基因下调表达,说明转录组测序结果可靠。
3. 讨论
植物细胞悬浮培养是一个持续变化的过程。在悬浮细胞培养过程中,接种量直接影响细胞的生长。细胞需要足够的空间和营养物质支持其增殖,选择适当的接种量对于细胞的生长至关重要。已有研究表明:落叶松胚性愈伤组织的最佳接种量为4 g·L−1[6]。油茶Camellia oleifera愈伤细胞悬浮培养的最佳初始接种量为33 g· L−1[23]。本研究中,胚性愈伤组织接种量为30 g·L−1,有利于香榧悬浮细胞的生长,此时细胞增长率最大。在细胞生长过程中,细胞内部很多起关键调控作用的酶对pH有一定的要求。陈继光等[24]研究表明:青钱柳在pH为5时细胞活力较强,细胞质量增量达最大,当pH达6时,细胞活力和质量增量都开始下降。本研究中,pH为5.7时,香榧胚性愈伤组织悬浮细胞抗逆性最强,细胞增长率最大。悬浮培养中摇床转速对细胞生长也有较大的影响。较低的转速会使愈伤组织堆积导致褐化[4];转速较高时会使细胞间产生碰撞导致破裂死亡,只有合适的转速才能使得细胞快速增殖生长[25]。本研究表明:转速为110 r·min−1时,香榧悬浮细胞生长最快。悬浮培养过程中对多种条件进行优化,能显著提升细胞的生长能力及抗性。
植物细胞在离体悬浮培养过程中,还须通过外施激素来促进细胞的生长。赤霉素在植物体的生命过程中起着重要的作用。当植物体处于胁迫条件时,外源赤霉素可以有效缓解胁迫的危害[26]。有研究表明:加入外源GA3可以有效缓解牡丹Paeonia × suffruticosa愈伤组织的褐化,并且提高愈伤组织诱导率[14]。本研究表明:在差异基因的GO富集分析中,GA3处理后上调表达基因主要参与催化活性、氧化还原过程、氧化还原酶活性等过程,说明活性氧的清除是香榧胚性愈伤组织增殖发育时的一个重要过程。在代谢通路中,差异表达基因主要富集在苯丙烷生物合成、淀粉和蔗糖代谢、木栓质合成等途径中。KEGG通路富集结果表明:苯丙烷生物合成途径中注释的差异表达基因最多,说明GA3处理后,香榧胚性愈伤组织的次级代谢途径产生了一定的变化。
苯丙烷代谢途径是植物体内多种防御性次生代谢产物(如黄酮类、木质素及水杨酸等)的主要来源[27]。有关木栓质相关合成途径的研究集中于植株根系以及部分受创伤部位[28],对于其在植物愈伤组织内的合成与作用研究较少。本研究中,木栓质合成途径也有7个差异基因被显著富集,且呈上调表达。已有研究表明:细胞色素P450单加氧酶(CYP)家族与FAR主要参与木栓质的合成[29]。木栓质大分子的酚类组分已证明主要有阿魏酸、香豆酸等物质,通过苯丙烷生物合成途径产生,可继续生成木栓质单体中相应的底物物质,在一定程度上促进了木栓质单体的合成,影响细胞生长,以应对环境的胁迫[30]。这与本研究结果相似,推测外源GA3处理下香榧愈伤组织苯丙烷生物合成途径所产生的酚类物质不仅参与了活性氧的清除,还参与了木栓质单体的合成,对愈伤组织的生长及胁迫适应性产生一定的影响[31]。
4. 结论
香榧胚性愈伤组织悬浮细胞生长曲线呈“S”型,接种后9~12 d为最佳继代时期,设置接种量为30 g·L−1,pH为 5.7,摇床转速为110 r·min−1,可获得生长良好的香榧胚性愈伤组织细胞,同时也可以提高悬浮细胞的抗氧化能力。通过转录组分析发现:外源GA3处理下香榧胚性愈伤组织细胞苯丙烷生物合成、木栓质生物合成等相关代谢通路为差异基因的主要参与部分,且其中部分调空愈伤组织形成、生长和发育的关键基因表达量产生变化,可能在香榧愈伤组织的生长发育、适应环境变化以及应对胁迫相关过程中发挥重要作用。
-
表 1 布局方案与可视栅格总数变化
Table 1. Layout scheme and the change of the total number of visible grids
i $ {\overline{{P}}}_{{i}} $ ${\left|{{V}}_{{i}}\right| }$ 0 6 1 972 1 6,29 3 677 2 6,29,36 4 737 3 6,29,36,12 5 664 4 6,29,36,12,26 6 384 5 6,29,36,12,26,18 6 767 表 2 属性集
Table 2. Attribute set
属性 属性名称 属性类型 yi1 增长监测面积 正向、收益、动态 yi2 交通情况 反向、成本、静态 yi3 坡度 反向、成本、静态 yi4 依附建筑情况 反向、成本、静态 表 3 基于DMACTSM迭代计算的综合属性值、选优和最终排序
Table 3. DMACTSM iterative computation
迭代次数 综合属性值 max(ui) 优建瞭望塔 a29 a26 a12 a6 a36 a18 0 0.76 0.30 0.50 0.68 0.35 0.29 0.76 $ {{a}}_{{29}} $ 1 0.56 0.45 0.55 0.47 0.44 0.56 $ {{a}}_{{26}} $ 2 0.54 0.51 0.36 0.50 0.54 $ {{a}}_{{12}} $ 3 0.54 0.33 0.48 0.54 $ {{a}}_{{6}} $ 4 0.53 0.47 0.53 $ {{a}}_{{36}} $ -
[1] 国家林业和草原局. 中国智慧林业发展指导意见[EB/OL].(2013-08-21)[2020-04-11]. http://www.forestry.gov.cn/main/72/content-623867.html. [2] ZHANG Kun, HUANG Zhi, ZHANG Songlin. Using an optimization algorithm to establish a network of video surveillance for the protection of Golden Camellia [J]. Ecol Inf, 2017, 42: 32 − 37. [3] GÖLTAS M, DEMIREL T, ÇAGLAYAN I. Visibility analysis of fire watchtowers using GIS: a case study in Dalaman State Forest Enterprise [J]. Eur J For Eng, 2017, 3(2): 66 − 71. [4] 黄贝. 基于模糊层次分析的森林防火瞭望台选址模型[J]. 林业建设, 2015(1): 19 − 23. HUANG BEI. Modeling the sitting of forest fire observatory based on fuzzy analytic hierarchy [J]. For Constr, 2015(1): 19 − 23. [5] 张健, 刘毅, 韩宁, 等. 鹫峰国家森林公园林火视频监控点的选址[J]. 东北林业大学学报, 2009, 37(5): 24 − 27. ZHANG Jian, LIU Yi, HAN Ning, et al. Site selection for video monitoring spots of forest fire in Jiufeng National Forest Park, Beijing [J]. J Northeast For Univ, 2009, 37(5): 24 − 27. [6] 中华人民共和国国家发展和改革委员会发展规划司.全国森林防火规划(2016-2025年)[EB/OL]. (2017-05-18). [2019-10-25]. http://www.ndrc.go-v.cn/fzgggz/fzgh/ghwb/gjjgh/201705/t20170518_847772.html. [7] 国家林业局计财司. 森林火情瞭望监测设施工程项目建设标准[EB/OL]. (2017-07-28). [2020-04-11]. https://max.book118.com/html/2017/1215/144197192.shtm. [8] 杨超. 北京市西山试验林场防火监测点布局研究[D]. 北京: 北京林业大学, 2006. YANG Chao. Study on Distribution of Monitoring Spots for Forestry Fire Protection on Beijing Xishan Forestry Farm[D]. Beijing: Beijing Forestry University, 2006. [9] 孙伟, 曹姗姗, 唐小明. 林火视频监控空间优化布局与评价技术[J]. 自然灾害学报, 2013, 22(2): 61 − 69. SUN Wei, CAO Shanshan, TANG Xiaoming. Spatial optimal arrangement and evaluation technology of forest fire video monitoring [J]. J Natural Disasters, 2013, 22(2): 61 − 69. [10] 肖化顺, 段祝庚, 邓立斌. 林区瞭望台监测网点选址研究[J]. 中南林业科技大学学报, 2012, 32(7): 6 − 9. XIAO Huashun, DUAN Zhugeng, DENG Libin. Research on obseravtion network setting in forest area [J]. J Central South Univ For Technol, 2012, 32(7): 6 − 9. [11] 孙传皓, 李贺洋, 郭少斌. 基于层次分析-模糊综合评判法的鄂尔多斯盆地东缘上古界泥页岩储层评判[J]. 地球科学前沿, 2015, 5: 43 − 52. SUN Chuanhao, LI Heyang, GUO Shaobin. Shale reservoir evaluation of upper paleozoic in the East Ordos Basin based on AHP-FCE method [J]. Adv Geosci, 2015, 5: 43 − 52. [12] 许水燕. 基于GIS的森林防火监测系统的研究与实现[D]. 南京: 南京农业大学, 2010. XU Shuiyan. Implementation Monitoring System in Forest Fire Prevention Based on GIS[D]. Nanjing: Nanjing Agricultural University, 2010. [13] 周成林, 颜伟, 姜霞. 浅谈山区森林防火监测网优化设计与实现[J]. 贵州林业科技, 2016, 44(4): 61 − 64. ZHOU Chenglin, YAN Wei, JIANG Xia. Brief talk about optimization design and implementation of forest fire monitoring network in mountainous areas [J]. Guizhou For Sci Technol, 2016, 44(4): 61 − 64. [14] 刘淑琼, 邹时林, 邹胜武, 等. 基于规则格网DEM提取山顶点的研究[J]. 东华理工大学学报(自然科学版), 2013, 36(增刊): 93 − 95. LIU Shuqiong, ZHOU Shilin, ZHOU Shengwu, et al. Extraction mountain peak based on grid DEM [J]. J East China Ins Technol, 2013, 36(suppl): 93 − 95. [15] 陈盼盼, 张友顺, 王春, 等. 基于DEM的山顶点快速提取技术[J]. 现代测绘, 2006, 29(2): 11 − 13. CHEN Panpan, ZHANG Youshun, WANG Chun, et al. Method of extraction surface peaks based on DEM [J]. Modern Surv Mapping, 2006, 29(2): 11 − 13. [16] 汤旭, 郑洁, 冯彦, 等. 云南省县域森林生态安全评价与空间分析[J]. 浙江农林大学学报, 2018, 35(4): 684 − 694. TANG Xu, ZHENG Jie, FENG Yan, et al. County-level forest ecological security evaluation and spatial analysis in Yunnan Province [J]. J Zhejiang A&F Univ, 2018, 35(4): 684 − 694. -

-
链接本文:
https://zlxb.zafu.edu.cn/article/doi/10.11833/j.issn.2095-0756.20190622






 下载:
下载:

 下载:
下载: